DETERMINATION OF THE FORM DRAG COEFFICIENTFOR FLAT-UNITS RAFTS

 

FedulovV.M., Barabanov V.A. (NAFU, Arkhangelsk, RF)

 

The article presents the results of the experimental research of the form drag coefficient of flat-units rafts.

 

Implementationofnewconstructionsoftimber raftsinindustrialpracticerequiresto knowledge of their hydrodynamic characteristics.Since theoretical investigations of the movement of rafts are extremely difficult even with modern computers, the research was carried out on the models in geometrical scale of 1:20. Previously performed studies have shown that such a scale is sufficient to proceed to full-scale size without taking into account the size effect[1].

Modeling was performed in the range of Froude numbers (0,017…0,095). Reynolds numbers varies in the range (0,5·10⁵…3,6·10⁵). Experimentswerecarriedoutatwatertemperature 15…16 °C. Towing of models was carried out at different speeds, corresponding to full-scale ranging from 0,35 to 1 m/sec.Length of the model varies from 0,65 to 2,00 m (from 13 to 40 m), draft – from 0,008 to 0,046 m (from 0,16 to 0,92 m), interval between flat units in length – from 0 to 0,05 m (from 0 to 1 m). Values corresponding to full-scale sizes are given in parentheses. Number of iterations in a series of experiments was taken as five, according to the recommendations [2]. In total, 190 series of experiments was performed.

Dependence for determining the resistance to motion of bodies in deep calm water in classic version is[3]:

.

(1)

Here – frictionaldrag; – formdrag; – frictiondragcoefficient; – frictionarea, ; – formdragcoefficient; – areaofmiddlesection, ; – waterdensity; – speed of uniform model motion;L, B, T – respectively, the length, width and draft of the raft.

Coefficientwas determined by the interpolated formula[4]:

.

(2)

Here–value of equivalentsandgrain roughness, forfull-scalerafts, formodels in geometrical scale of 1:10 – 1:50[5].

Formula (4) is applicable to a range of . Our range gets into these limits.

Coefficientwas defined as:

.

(3)

Total resistance R was determined by the results of experimental studies, depending of the speed of uniform model motion, length, draft, intervals between flat units in the length and width. For this purpose experiments were carried out by towing the model at 5 different speeds. Here for each speed we determined the total resistance R. Then we built the graphs of the total resistance R on the speed of towing . Three of these graphs for different models are presented in Figure 1.

Figure 1 – Graphs of the total resistance on the speed of towing

 

These graphs allowus to determine total resistance depending of the speed of model motion for a particular model of raft. For example, Figure 1 shows plots for the models ofrafts, composed of 4 (5AC2), 8 (5AC4) and 12 (5AC6) flat units.

To estimate the relationship between values obtained by experimental and theoretical dependence, determination coefficient D was compute. In our case D = 0,99.

After the calculation of coefficients  for each flat-units raft model, the approximation functions were picked up by the least-squares method according to the following forms:

if; if; if; if; if.

(4)

 

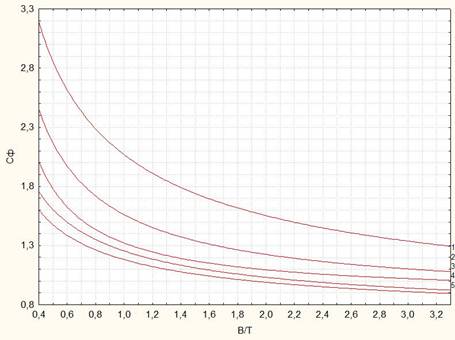
Figure 1 – A plot of the form drag coefficient on the ratio of 

 

Dependencies (4) applytofull-scalerangeofspeedsfrom 0,35to 1 m/sec, forarangeoftheratio.Figure 1 shows the graphs constructed from formulas (4). Line 1 correspondstotherangeof Froude numbers (0,017…0,025), line 2 -, line 3 - , line 4 - , line 5 - .

Knowing the form drag coefficients for flat-units rafts will perform more efficiently transport and process calculations while working on new timber rafting technologies. For example, if we know form drag coefficient, we can calculate the total resistance of the water, and consequently to pick up the tugboat for raft transportation.

References

1.Мурашова О.В. Исследование гидродинамических характеристик плоских сплоточных единиц на моделях и в натурных условиях / О.В. Мурашова, А.А. Митрофанов // Изв.вузов. Лесной журнал №1, 2007 - С. 58-66.

2. Митрофанов А.А. Лесосплав. Новые технологии, научное и техническое обеспечение. / А.А. Митрофанов //Монография. - Арх-ск :Изд-во АГТУ, 2007. - 492 с.

3. Павленко Г.Е. Сопротивление воды движению судов / Г.Е. Павленко. - Москва: Морской транспорт, 1956. - 508 с.

4. SchlichtingH. Boundary-layertheory. / H. Schlichting, K. Gersten–Berlin-Heidelberg-N.Y.: Springer-Verlag, 2000. - 795p.

5. Худоногов В.Н. Гидродинамическое взаимодействие плотов и внешней среды. / В.Н. Худоногов. – Красноярск: Красноярское книжное издательство, 1966. - 225 с.